A Critical Review of Turbulence Characteristics in Open Channel Flow

Abstract

This study subject encompasses a comprehensive evaluation of the available literature on turbulence in open channel flows. Open channel flows are characterized by intricate fluid dynamics, including the production of turbulent eddies and vortices that can profoundly alter transport processes and mixing of diverse fluids. The paper addresses the numerous forms of turbulence that can occur in open channels, such as coherent structures, secondary currents, and bed-generated turbulence. We investigate the elements that determine the intensity and features of these turbulent flows, including channel geometry, flow velocity, fluid properties, and roughness of the channel substrate. The paper also assesses the current theoretical models used to characterise open channel turbulence and the experimental techniques used to quantify and demonstrate turbulent flows in open channels. By critically scrutinising the strengths and limits of the current literature, this review outlines the essential research gaps that need to be addressed to expand our understanding of turbulent open channel flows. This study contributes to the development of enhanced models and techniques for managing and modulating open channel flows in diverse applications.

Introduction

I. INTRODUCTION

The complicated phenomenon known as open-channel flow turbulence has been intensively explored for many years. In open channels like rivers, canals, and streams, the fluid interacts with the free surface and the bed to generate this form of flow. There are still a lot of unresolved problems and challenges that need to be addressed despite significant inquiry. The study of open-channel flow turbulence has entered a new phase in the twenty-first century owing to the swift improvement of computational and experimental approaches. The objective of this study is to explore the most recent developments in our knowledge of the science of open channel flow turbulence and the applications of it in Hot-wire anemometers that have been employed in airflow experiments since the 1950s to study turbulent boundary layers and duct flow. In 1980s the laser anemometry has substantially lowered the difficulties of undertaking the research in open channel turbulence, permitting in-depth analyses of unstable and channel flows as well as more basic two-dimensional uniform flows. It is crucial to grasp open-channel flow turbulence for managing water resources, controlling floods, and conserving the environment.

A. Importance of Shear Stress and Eddy Vorticity

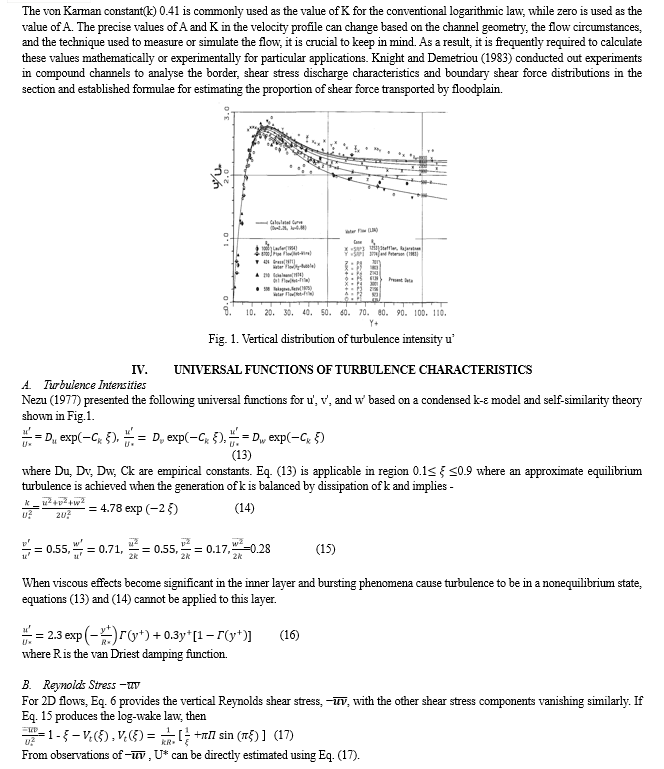
One of basic term in the study of fluid mechanics is shear stress which is the force per unit area that the fluid exerts on the boundary surface. In the flow of an open channel, the fluid's momentum is transmitted to the bed and the free surface by the shear stress. The degree of bed erosion, sediment movement, and the formation of bedforms like ripples and dunes are all impacted by the intensity of the shear force. Shear stress is another key aspect in the design of hydraulic structures like weirs, spillways, and energy dissipators, particularly when it comes to their stability and size. Using the depth-integrated Navier-Stokes equation, they provided an analytical solution that took into account the effects of bed friction, lateral turbulence, and secondary currents. Tang and Knight (2008) have devised a technique for evaluating average depth velocity and distribution of shear stress for overbank flows in compound channels using depth integrated Navier Stokes equation. They propose an analytical solution that addresses the effects of bed friction, lateral turbulence, and secondary currents. The existence of secondary current close to the interface area has been hypothesised by Carter and Williams (2008). They demonstrated the presence of a persistent secondary flow at the internal corner that exacerbates bed stress on the floodplain. In order to anticipate the stage-discharge correlations in compound channels with greater width ratios. Khatua and Patra (2012a and 2012b) developed an innovative method called MDCM and reported apparent shear stress in terms of interaction length. Rouse, H. (1937) proposed that turbulence is a random, chaotic motion that arises in fluids when the flow is disrupted. He argued that turbulence is characterised by the occurrence of eddies, which are zones of spinning fluid that mix the fluid and carry momentum and energy throughout the flow.

II. TURBULENCE MODELS

Turbulence models are commonly applied in computational-fluid-dynamics (CFD) simulations to predict flow behaviour in open channels. Open channel flow is characterised by free surface and shear flow, and turbulence models are required to effectively capture the intricate flow dynamics. Chow, V. T. (1959) assesses the limitations and advantages of each model and makes ideas on selecting the correct model for a certain application. Yen, B. C. (1977) also investigated the theories and models used to characterise turbulence, including the Kolmogorov theory, which specifies the behaviour of small-scale turbulence, and the Reynolds stress model, which characterises the transfer of momentum in turbulent flows.

A. Large Eddy Simulation (LES) method

LES is a numerical technique that solves a filtered version of the Navier-Stokes equations. This filtered representation retains the large-scale patterns of the flow, while the small-scale structures are approximated using sub grid-scale models. LES is less computationally demanding than DNS and allows for the modelling of turbulent flow over lengthy periods of time. It also gives the ability to examine the impact of roughness on the turbulent flow. Yet, LES still takes large computer resources and the choice of sub grid-scale model could influence the conclusions. Thomas and Williams (1995) presented a large eddy simulation model for a constant Reynolds number of 430,000 in a typical trapezoidal shaped compound channel of trapezoidal shape with a steady, uniform flow. It was researched how the main canal and the flood plains interacted. Numerical modelling was utilised to determine the distribution of bed shear stress, velocity dispersion, and secondary current circulation over the flood plain. Experimental data were gathered at the Hydraulics Research Ltd. SERC Flood Channel Facility in Wallingford, UK. These experimental data were contrasted with the outcomes of the LES simulation. Salvetti et al. (1997) conducted LES for a uniform and steady flow in a compound channel of regular shape with a substantial Reynolds number and got the distribution of bed shear stress, secondary motion and vortices compared with the observed data. Sandeep Bomminayuni and Thorsten Stoesser (2011) describes the findings of an LES of turbulent flow over a channel bed intentionally roughened by hemispheres. . First and second order statistics are compared with matching laboratory experiments to validate the LES.

B. RANS Approach

The Reynolds averaged Navier Stokes turbulence model is a commonly used approach for simulating turbulent flow in fluid mechanics. In this model, the time averaged Navier Stokes equations are used for calculation of the mean flow properties, while the turbulence is modelled using additional equations for the turbulence quantities. RANS turbulence models are frequently categorised based on how many extra differential transport equations are needed to calculate the turbulence variables.

In RANS models, the turbulent stresses are split into a mean part and a fluctuating component, and the Reynolds stresses are approximated using empirical relationships. The most commonly used RANS models are the standard k-ε model, the realizable k-ε model, and the k-ω model. In the standard k-ε model, the turbulence kinetic energy (k) and its dissipation rate (ε) are modelled using transport equations. The model assumes a two-equation system, where k represents the energy of the turbulent eddies and ε represents the rate at which the turbulent kinetic energy is dissipated into heat. The model is based on the assumption that the turbulence is isotropic and homogeneous. The k-ω model is another commonly used RANS model that models the turbulence using the turbulence kinetic energy and the specific dissipation rate of the turbulent kinetic energy. The model is based on the assumption that the turbulence is locally isotropic and homogeneous. Bradshaw et al. (1967) used a model to calculate the velocity profiles in boundary layers that only requires a differential transport equation for the turbulent kinetic energy.

C. Law of the Wall

The behaviour of turbulent flow close to a solid boundary is described by the law of the wall, a fundamental principle in fluid dynamics. Jiménez, J., & Moin, P. (1991) In the paper, Jiménez and Moin claim that the behaviour of turbulence near barriers may be characterised by a "minimum flow unit" (MFU), which is a self-similar, streamwise-aligned structure that occurs at the lowest scales of motion. The MFU is regarded to be responsible for the transfer of momentum and energy in the near-wall region and has a vital role in determining the frictional drag on surfaces. German physicist and engineer Ludwig Prandtl made the initial suggestion in 1925. Townsend, A. (1951) also created the idea of the "inner and outer regions" of a turbulent boundary layer. He argued that the flow near a solid barrier is dominated by small-scale turbulence, while the outer section of the flow is dominated by larger-scale turbulence. Wood and Bradshaw (1984) discovered that the side wall impacts the turbulence inside the plane mixing layer, prior and afterwards the mixing layer reaches the wall. According to the law of the wall, the fluid's velocity rises in turbulent flow close to a solid boundary in proportion to the logarithm of the distance from the boundary.

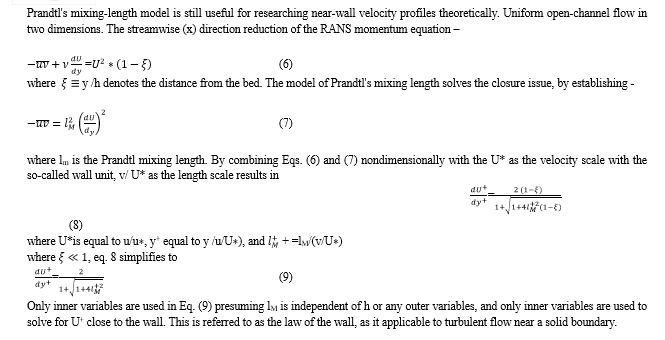
III. THE BEGINNINGS OF EXPERIMENTATION IN TURBULENT OPEN-CHANNEL FLOWS

A. Methods for Measuring Open-Channel Turbulence

The uneven and unpredictable motion of fluid in open channels like rivers, streams, and canals is referred to as "open-channel turbulence." Understanding and predicting the flow of sediment and contaminants, as well as constructing and maintaining hydraulic structures, depend on measuring open-channel turbulence. Below are a few methods used to measure turbulence in open channels. (1) Hotwire Anemometry: In hotwire anemometry, the velocity of fluid particles in the channel is measured by heating a thin wire with an electric current. Although this method is extremely accurate and capable of producing high-frequency velocity readings, it can only be used for moderately slow flows. (2) Laser Doppler Velocimetry (LDV) is a non-intrusive method for determining the speed of fluid particles moving through a channel. This method is expensive and requires specialist equipment, but it is quite accurate and can produce detailed velocity profiles. (3) Particle Image Velocimetry (PIV)-PIV is a unintrusive technology that uses laser light to take pictures of the particles as they move through the channel after seeding the flow with microscopic particles. Following that, velocity vectors and turbulence statistics can be computed using these images. PIV is very precise and capable of providing extensive details about turbulence structures, but it is also costly and calls for specialist equipment. (4) Acoustic Doppler Velocimetry (ADV): ADV measures the velocity of fluid in a channel using sound waves. High-frequency velocity measurements can be obtained with this technique, but it is less accurate than LDV or PIV. (4) Pressure sensors can be employed to gauge pressure changes brought on by turbulence in a channel. Then, turbulence statistics like Reynolds stress and turbulent kinetic energy can be computed using these measurements. Although they are reasonably priced and can give a decent approximation of turbulence intensity, pressure sensors cannot give precise information about the structures of turbulence. (5) Fluctuations in Surface Elevation: Fluctuations in Surface Elevation can be used to calculate the channel's turbulence strength. In this method, the surface elevation is measured several times along the channel, and the standard deviation of these readings is computed. Although this approach is straightforward and reasonably priced, it does not reveal the turbulence structures or velocity profiles.

B. Review of Turbulence Measurements in Water

In a study by researchers from, Ippen and Raichlen (1957) examined the properties of wind-generated waves in a laboratory flume. The goal of the study was to comprehend how wind speed relates to wave parameters like wavelength and height. Researchers used a total-head tube along with a pressure transducer to quantify turbulence features, but a total-head tube is unable to discern between variations in velocity and variations in pressure Bigillon et al. (2006) found the wall similarity assumption for transitionally rough wall scenarios by studying the turbulence characteristics of open channel flow.

Nakagawa et al. (1975) developed a unique technology using a two-component hot film probe for measuring the 3D flow for flowing open channel through smooth and coarse beds. The findings of analogous investigations in boundary layers and pipelines that employed HWA were then compared. To determine the fluid's velocity, first introduced the concept of LDA in the 1960s. Y. F. Chang and H. J. Adrian at the University of Michigan created the first usable LDA system in the late 1960s. This method produced two laser beams that were concentrated into a small amount of fluid using a helium-neon laser. Photomultiplier tubes were used to detect a Doppler shift caused by the laser light being dispersed by fluid particles. The Doppler shift of the dispersed light can be used to calculate the fluid's velocity. LDA has taken HFA's place as the preferred method for researching turbulence in water flows since the 1990s. In their study, Mohamadian, Khojastehzadeh, and Kianpisheh (2021) offer a substantial addition to our understanding of turbulent flow and its interaction with structures. This insight has repercussions for the building of more exact and trustworthy models for use in engineering design and analysis.

C. Classification of 2D and 3D Open-Channels

Researchers started concentrating on the variations between 2D and 3D open-channel flow in the middle of the 20th century. Ackers and White's work, which presented a classification system based on the ratio of the flow depth to the channel width in 1973, was one of the early research in this field. They designated 2D channels as those with a flow depth to channel width ratio under 0.1 and 3D channels as those with a ratio over 0.1. Significant advancements in our understanding of 3D open-channel flow were made by Nezu and Rodi (1985). In their investigation, the three elements of velocity (u, v, and w) were measured in a lab flume under various flow scenarios. Also, they created a mathematical model to forecast Reynolds stresses in 3-D open-channel flows, a crucial quantity for defining turbulence. Nezu and Nakagawa (1993a) proposed the b/h ratio as a parameter for characterizing the secondary currents in 3D flows in open channel flows.

D. Exploring the Significance of Inner and Outer Layer Theory in Characterizing Turbulence Dynamics in Open Channels

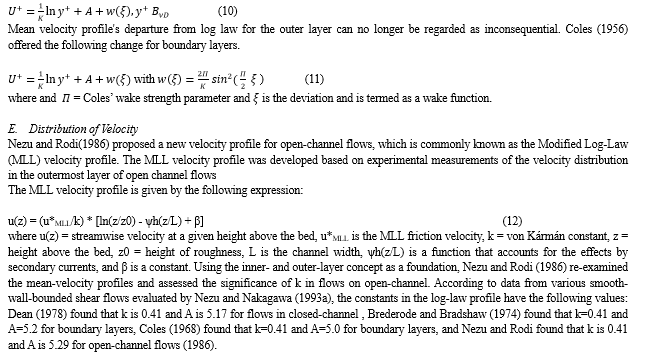
An essential idea in fluid dynamics, the inner- and outer-layer theory is particularly pertinent to the investigation of turbulent flows. The theory offers a framework for comprehending the composition of turbulent boundary layers, which are frequently seen in numerous engineering and environmental applications. The law of the wall applies only to the inner layer, and the mean velocity profile distant from the beds deviates from the log-law. Keulegan (1938) stated that the logarithmic law may be taken into account for velocity distribution throughout the entire depth of flow in open channels.

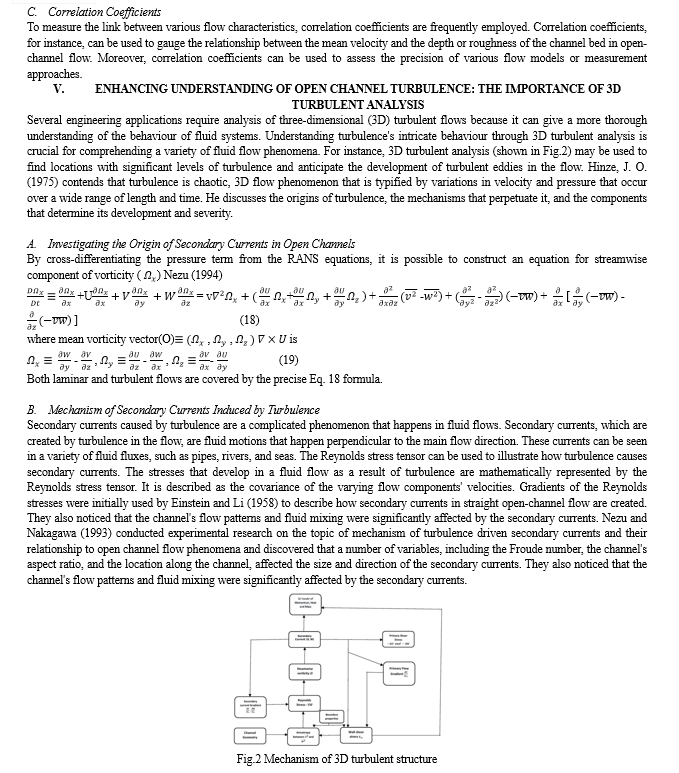
VI. PROSPECTS FOR ADVANCED OPEN-CHANNEL TURBULENCE RESEARCH IN 21ST CENTURY FLOW DYNAMICS

1. Simulation using large-eddy theory (LES): As computer capacity has increased, LES has gained popularity as a method for modelling complex turbulent flows, including open-channel flows. Enhancing LES models for open-channel flows in the future may be the main goal in order to better depict the complex interactions between secondary currents, bed topography, and atmospheric boundary layer.
2. Advanced Measurement Techniques: Researchers have been able to collect highly detailed data on intricate open-channel flows because to advancements in measuring techniques like PIV, planar laser-induced fluorescence (PLIF), and ADV. The goal of future study may be to combine these methods in order to assess the velocity, turbulence, and transport characteristics of open-channel flows more thoroughly.
3. Sustainable Water Resources Management: It is necessary to create sustainable management plans for rivers, estuaries, and coastal areas since population increase, urbanisation, and climate change are placing more strain on water resources. Future studies might concentrate on developing sophisticated open-channel turbulence models to improve various methods for managing water resources, including flow control devices, river restoration, and water quality control.
4. Multiscale Modelling: A multiscale phenomenon, open-channel turbulence occurs over a variety of length and temporal scales. The development of multiscale models that can depict interactions between large-scale structures and small-scale turbulent eddies, as well as the impacts of turbulence on the processes of sediment transport and erosion, may be the main focus of future research.

Conclusion

Open-channel flow turbulence is a complicated and dynamic phenomenon with significant practical ramifications for hydraulic engineering, environmental protection, and management of water resources. Throughout the past few decades, breakthroughs in experimental and numerical methodologies have significantly advanced our understanding of open-channel flow turbulence. The potential for considerable improvements in our knowledge of the dynamics of open-channel flows and their practical applications makes the research prospects for complicated open-channel turbulence in the twenty-first century very promising. Future studies might concentrate on developing more precise turbulence models, better understanding the relationships between primary and secondary currents, and the effects of turbulence on sediment transport and erosion processes. Moreover, open-channel flow turbulence research has important ramifications for managing water resources sustainably, including river restoration, flow control structures, and water quality. The issues brought on by population increase, urbanisation, and climate change will be addressed in large part by advancements in turbulence research in the twenty-first century.

References

[1] AT Ippen, F Raichlen (1957) Journal of the Hydraulics Division doi-10.1061/JYCEAJ.0000127 [2] Bigillon et al. (2006) Measurements of turbulence characteristics in an open-channel flow over a transitionally-rough bed using particle image velocimetry doi: 10.1007/s00348-006-0201-2 [3] Bousmar et al. (2005) Upstream Discharge Distribution in Compound-Channel Flumes doi:10.1061/(ASCE)0733-9429(2005)131:5(408) [4] Camenen et al. (2006) Equivalent Roughness Height for Plane Bed under Steady Flow doi:10.1061/(ASCE)0733-9429(2006)132:11(1146) [5] Carter and Williams (2008). Large eddy Simulation of a long asymmetric compound open channel. Journal of Hydraulic Research,46(4),445-453. [6] Chow, V. T. (1959). Open-channel hydraulics. McGraw-Hill. doi: 10.1002/qj.49708536511 [7] Einstein, H. A., and Li, H. (1958) “Secondary currents in straight channels,” Trans. AGU, 39, 1085–1088. [8] Hinze, J. O. (1975). Turbulence. McGraw-Hill. doi: 10.1007/978-1-4684-8239-9 [9] Jiménez, J., & Moin, P. (1991). The minimal flow unit in near-wall turbulence. Journal of Fluid Mechanics, 225, 213-240. doi: 10.1017/S0022112091002502 [10] Keulegan (1938) LAWS OF TURBULENT FLOW IN OPEN CHANNELS, Part of Journal of Research of the National Bureau of Standards, Volume 21 [11] Khatua and Patra (2012) Stage-Discharge Prediction for Straight and Smooth Compound Channels with Wide Floodplains doi.10.1061/(ASCE)HY.1943-7900.0000491 [12] Knight and Demetriou (1983) Flood Plain and Main Channel Flow Interaction doi:10.1061/(ASCE)0733-9429(1983)109:8(1073) [13] Knight and Demetriou (1983) Flood Plain and Main Channel Flow Interaction doi:10.1061/(ASCE)0733-9429(1983)109:8(1073) [14] Knight and Hamed (1984) Boundary Shear in Symmetrical Compound Channels doi:10.1061/(ASCE)0733-9429(1984)110:10(1412) [15] Knight and Shiono (1990) Turbulence measurements in a shear layer region of a compound channel doi:10.1080/00221689009499085 [16] Mohammadian, M., Khojastehzadeh, M., & Kianpisheh, S. (2021). Spatially varying bed roughness effects on open channel turbulence. Journal of Hydro-environment Research, 30, 235-246. doi: 10.1016/j.jher.2021.01.007 [17] Nakagawa, H., Nezu, I., and Ueda, H. (1975). “Turbulence of open channel flow over smooth and rough beds.” J. JSCE, 241, 155–168 [18] Naot, D., Nezu, I., and Nakagawa, H. (1993). “Hydrodynamic behavior of compound rectangular open-channels.” J. Hydraul. Eng., 119(3), 390–408 [19] Nezu (2005) “Journal of Hydraulic Engineering”.doi: 10.1061/ (ASCE)0733-9429(2005)131:4(229) [20] Nezu and Nakayama (1997) Space-time correlation structures of horizontal coherent vortices in compound open-channel flows by using particle-tracking velocimetry doi:10.1080/00221689709498426 [21] Nezu, I. (1977). “Turbulent structures in open-channel flows.” PhD thesis, Kyoto Univ., Japan [22] Nezu, I., & Nakagawa, H. (1993). Turbulence in open-channel flows. A.A. Balkema. doi: 10.1201/9781439835291 [23] Nezu, I., and Nakagawa, H. (1989a). “Turbulent structure of backwardfacing step flow and coherent vortex shedding from reattachment in open-channel flows” Turbulent shear flows 6, Vol. 6, Springer, NewYork, 313–337. [24] Nezu, I., and Rodi, W. (1985). “Experimental study on secondary currents in open channel flow.” Proc., 21st IAHR Congress, Vol. 2, Delft, The Netherlands, 114–119 [25] Nezu, I., and Rodi, W. (1986). “Open-channel measurements with a laser Doppler anemometer.” J. Hydraul. Eng., 112(5), 335–355. [26] open-channel flows.” Turbulent shear flows 6, Vol. 6, Springer, New York, 313–337. [27] Rajaratnam, N. (1976). Turbulent jets and plumes. Elsevier. doi: 10.1016/B978-0-444-41710-9.50004-9 [28] Rezaei and Knight (2009). Application of the Shiono and Knight Method in compound channels with non-prismatic floodplains.doi.10.3826/jhr.2009.3460 [29] Rodi, W. (1980). Turbulence models and their application in hydraulics, IAHR-Monograph, Balkema, Rotterdam, The Netherlands [30] Rouse, H. (1937). Modern conceptions of turbulence. Transactions of the American Society of Civil Engineers, 102, 1-46. doi: 10.1061/TACEAT.0007091 [31] Salvetti, Zang, Street and Banerjee (1997). Large-eddy simulation of free-surface decaying turbulence with dynamic subgrid-scale models, doi: 10.1063/1.869359 [32] Sandeep Bomminayuni1 and Thorsten Stoesser (2011) Turbulence Statistics in an Open-Channel Flow over a Rough Bed doi:10.1061/(ASCE)HY.1943-7900.0000454 [33] Tang and Knight (2008). Lateral Depth-Averaged Velocity Distributions and Bed Shear in Rectangular Compound Channels, doi.10.1061/(ASCE)0733-9429(2008)134:9(1337) [34] Thomas and Williams (1995). Large eddy simulation of turbulent flow in an asymmetric compound open channel. doi.10.1080/00221689509498682 [35] Tominaga, A., and Nezu, I. (1991). “Turbulent structure in compound open-channel flows.” J. Hydraul. Eng., 117(1), 21–41. [36] Townsend, A. A. (1951). The structure of turbulent shear flow. Cambridge University Press. doi: 10.1017/CBO9780511564345 [37] Wood and Bradshaw (1984) A turbulent mixing layer constrained by a solid surface. Part 2. Measurements in the wall-bounded flow doi:10.1017/S0022112084000392 [38] Yen, B. C. (1977). Open-channel flow turbulence. Journal of Hydraulic Research, 15(1), 1-21. doi: 10.1080/00221687709499676

Copyright

Copyright © 2023 Siprarani Pradhan, Lokesh Dash, Kishanjit Kumar Khatua. This is an open access article distributed under the Creative Commons Attribution License, which permits unrestricted use, distribution, and reproduction in any medium, provided the original work is properly cited.